CSC/ECE 506 Spring 2010/ch 3 yl

Supplement to Chapter 3: Support for parallel-programming models. Discuss how DOACROSS, DOPIPE, DOALL, etc. are implemented in packages such as Posix threads, Intel Thread Building Blocks, OpenMP 2.0 and 3.0.

Parallel-programming models

Loop-independent vs. loop-carried dependences

Before performing the three kinds of parallelism analysis, we need to discuss about loop-dependence analysis first.

Statement dependences

To discuss code analysis, let's define the framework as follows. Let S denote a statement in the source code. Let [] denote loop iteration space. Let S1 -> S2 denote statement S1 executes before S2. Then, we further define three statement dependences:

• S1 ->T S2 denotes true dependence: S1 -> S2, and S1 writes to a location that is read by S2.
• S1 ->A S2 denotes anti dependence: S1 -> S2, and S1 reads a location written by S2.
• S1 ->O S2 denotes output dependence: S1 -> S2, and S1 writes to the same location written by S2.

To give a better understanding, we illustrate the dependences using the code section below:

for (i=1; i<N; i++)
    S1: a[i] = i;
    S2: b[i] = a[i-1];
    S3: b[i] = a[i]+c[i+1];
    S4: c[i] = 2*i;

The dependences corresponding to the code are:

• S1[i-1] ->T S2[i]: a[i-1] is written in statement S1 in the (i-1)th iteration, and read in S2 in the ith iteration.
• S1[i] ->T S2[i]: a[i] is written in statement S1 in the ith iteration, and read in S3 in the ith iteration.
• S3[i] ->A S4[i]: a[i] is read in statement S3 in the ith iteration, and written in S4 in the ith iteration.
• S2[i] ->O S3[i]: b[i] is written in statement S2 in the ithe iteration, and also written in S3 in the ith itertation.

DOALL

for    i:=2:N-1 do A(i):=[A(i-1) + A(i) + A(i+1)]/3; next i;
forall i:=2:N-1 do A(i):=[A(i-1) + A(i) + A(i+1)]/3;

for (i=2; i<=n; i+=2)
 s: a[i] = a[i-2];
for (i=3; i<=n; i+=2)
 s: a[i] = a[i-2];

DOACROSS

In code 3.7, apparently there is a loop-carried dependence existing in the code.

S[i] -> T S[i+1]

Obviously, there is no way to implement it as DOALL parallelism.

Code 3.7 A loop with loop-carried dependence
for (i=1; i<=N; i++) {
 S: a[i] = a[i-1] + b[i] * c[i];}

If we split code 3.7 as two loops, then the fist loop can be implement in DOALL parallism. In code 3.8, first of all, we create a new array named temp[i]. Second, we put temp[i] in a loop which is individual and loop-independence. However, this solution generated a disadvantage: high storage. Due to increasing the array size of temp by i++, the size of temp depends on the number of iterations instead of threads. If N ( # of iteration) is bigger, then the size of temp will be larger.

Code 3.8 A split version of the loop in Code 3.7
for (i=1; i<=N; i++) {  //this loop has DOALL parallelism
  S1: temp[i] = b[i] * c[i];}  
for (i=1; i<=N; i++) {
  S2: a[i] = a[i-1] + temp[i];}

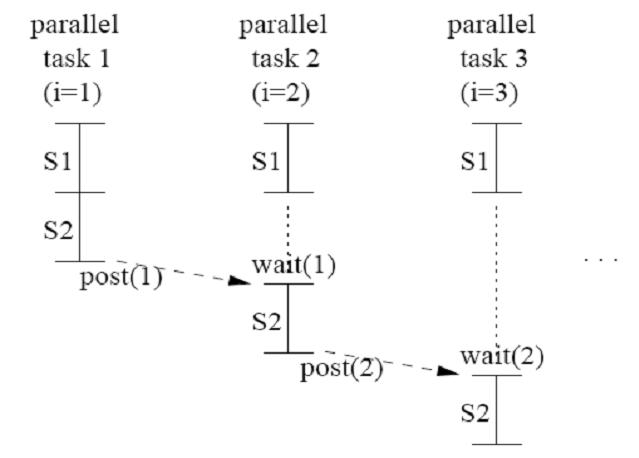
Let's see code 3.9, We still create a new array temp[i]. There are two differences between code 3.9 (DOACROSS) and code 3.8 (DOALL). First, the size of array is not increasing by the # of iterations but the # of threads, because temp[i] is not a shared array but a private scalar. Second, there is only one loop which contains S1 and S2. In DOACROSS parallelism, we insert two primitives, wait(name) and post(name). The principle of DOACROSS parallelism is to insert point-to-point synchronization. Notice the statement post(i) and and wait(i-1). post(i) is a signal produced by producer a[i]. Once a[i] has been produced, it will be post immediately. Meanwhile, the consumer is waiting i-1 by using wait(i-1). The reason makes consumer has to wait the previous a[i] is because S2 has to use a[i-1] to calculate to generate a[i]. Next, when S2 produce a[i], it will post it, post(i), to let the next consumer consume it. We will discuss how DOACROSS parallelism works in figure later.

Code 3.9 Exploiting DOACROSS parallelism in the loop from code 3.7
post(0);
for (i=1; i<=N; i++) {
  S1: temp[i] = b[i] * c[i];}
  wait(i-1);
  S2: a[i] = a[i-1] + temp[i];
  post(i);}

References

1. wikipedia: Parallel Computing
2. FUNDAMENTALS OF PARALLEL COMPUTER ARCHITECTURE, Yan Solihin, Aug 2009